Facts about Napier’s Bones
- Napier’s Bones was one of several methods John Napier invented to simplify calculating large numbers.
- Napier’s Bones and other calculating techniques were published in 1617 in a book titled Rabdologiae, or speaking rods, shortly before Napier’s death.
- Napier’s Bones were in use for hundreds of years, until mechanical calculators were invented.
Napier’s Bones History
Napier’s Bones is a manual calculating device using strips of ivory or other types of material that are divided into sections. The sections are marked with numbers or digits and are used primarily for multiplication and division. This particular method had its origins in lattice multiplication. The purpose is for quickly finding quotients and products of numbers.
John Napier invented what is known as Napier’s bones and it was published in 1617. John Napier was from Merchiston, Scotland. He called his invention “rabdology.” Napier also published the book in the same year with the title Rabdologiae. He was also the first to use decimal points and binary numbers in mathematical calculations.
Quick Facts
- Created
- 1617
- Creator
- John Napier
- Original Use
- To simply multiplication and division.
- Cost
- Unknown
Napier designed the bones because he was frustrated with the tedious and error-prone process of working with large numbers. He was particularly hoping to ease his own difficulties when calculating logarithmic tables. He had invented logarithms in 1614.
He decided to publish his work regarding the bones because so many of his friends, to whom he had shown the numbering rods, were so pleased with the method that they were already becoming widely used. They were even being used in other countries.
The Rabdologiae had four parts to the book, two basic parts and two appendixes:
- Book I describes the calculating tool.
- Book II includes forty-seven pages of examples, tables, and general problems.
- Book III is an appendix on Napier’s promptuary, another calculating device.
- Book IV is an appendix devoted to location arithmetic.
Napier’s Bones: How It Worked
Napier’s Bones are numbered rods to help work different multiplication problems. Multiplication tables are embedded within a series of rods. There are a variety of designs for the bones or rods. The rods can be made of wood, ivory, or metal. They are sometimes made of heavy cardboard or plastic. Besides multiplication, Napier’s bones supports calculation for division, addition, subtractions, and square root.
Each individual rod has four faces with a multiplication table engraved on each one. This enables the multiplication problems to be completed more simply as addition problems, and division problems as subtraction operations. There are ten rods, or bones, that correspond to each digit, 0 through 9. An eleventh bone is for representing the multiplier.
A multiplier rod is a list of the numbers 1 through 9 that are arranged downward in a vertical fashion. Several sets of bones were used for multiplication with numbers that contained repeating digits.
Napier called the first digit a “single.” The ongoing squares have multiples of the single. Twice the single, and then three times the single, and so forth until the process goes through the ninth square. Therefore, the ninth square has nine times the number at the very top square. There are also ways of using the rods to extract square roots.
The device normally involves several parts. This includes the base board and a rim where Napier’s rods are put inside to carry out each multiplication or division problem. The left edge of the board is divided into nine areas or squares.
Every square has a diagonal line from the left bottom corner up to the right corner. This divides each square, except for the very top, equally in half. Each of these areas has the numbers 1 through 9. Each set of bones may be designed within a carrying case..
The following is a diagram of what Napier apparently based his invention on. This method was called gelosia (jealousy) in Italian. This particular example shows how to multiply 456 x 128. The answer along the lower left corner is 58,368.
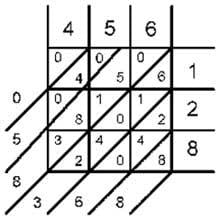
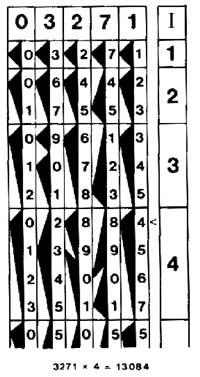
The following is another drawing, demonstrating how to solve the problem 3105 x 6. After arranging side by side four rods (for 3, 1, 0 and 5), we should take the row for the factor 6 (marked with arrow). We start from the right, taking initially zero from the lower right part of the first cell. It will be the first digit of the result.
Then we have to add the three digits from the left part of the first cell with 0 from the right part of the second cell. Continuing to add the digits along the diagonals, and we will get the proper result 18630.
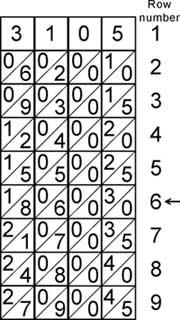
The next drawing explains the multiplication of a number, which has identical digits. For this we have to use identical rods. The four faces of each rod contain multiples of one of the nine digits, and is similar to one of the slips just described.
The first rod contains the multiples of 0, 1, 9, 8, the second of 0, 2. 9, 7, the third of 0, 3, 9, 6, the fourth of 0, 4, 9, 5, the fifth of 1, 2, 8 , 7, the sixth of 1, 3, 8, 6, the seventh of 1, 4, 8, 5, the eighth of 2, 3, 7, 6, the ninth of 2, 4, 7, 5, and the tenth of 3, 4, 6, 5. Each rod, therefore, contains on two of its faces factors of digits which are complementary to those on the other two faces, and the factors of a digit and its complement are reversed in position.

.
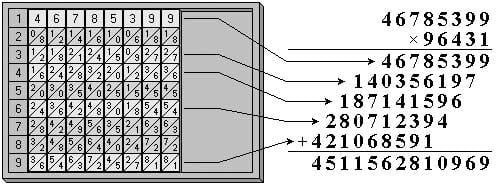
The next figure demonstrates when the second factor is multidigital, that intermediate products must be written manually, shifting one position leftwards, then intermediate products must be added. After arranging rods for the multiplicand side by side, we have to multiply the multiplicand (46785399) by the units of the multiplier (96431).
The result is 46785399×1=46785399. Then we have to multiply the multiplicand by the tens, the multiplier 46785399×3=140356197, shifting the result one position left and to continue, while all digits of the multiplier will be used. Then we have to add manually the partial factors.
Eventually, an adding machine in which multiplication can be done without any thinking was developed some years later by Wilhelm Schickard, who used Napier’s Rods in his Rechenuhr (Calculating Clock.)
The use of Napier’s rods is easy, but tedious when one wants to multiply two numbers each having two or more digits. That’s why Napier went further, and in book III of Rabdologiae he described a more elaborate calculating device, consisting of engraved rods and strips. On the following figure to the left is placed the thick rod for the digit 4, to the right is placed a thin rod for the 7, cut off (missing) triangles are filled with black color.
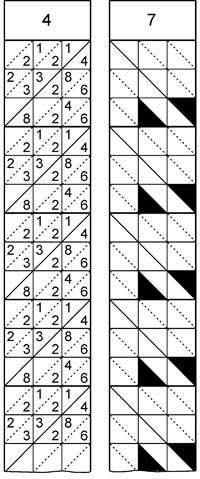
The following is an example using tick rods that are filled with digits (or empty for zero). Squares of the rods, which have digits 1-9 (not 0), are divided into 9 smaller squares, and each of these squares is divided in half into 3 triangles by means of a diagonal, parallel to the diagonal in the bigger square. In the triangles are inscribed digits according to the letters a, b, c, d, e, f, g, i, j.
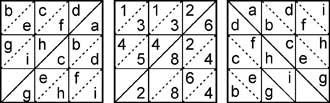
In the following figure you can see the multiplication 7213×524. First, there must be arranged properly thick rods, according to the multiplicand 7213. Then the thin rods, according to the multiplier 524, are placed over and rotated to the 90 degrees to the thick, with the diagonals of squares on the thick and thin rods to be concurrent. The result can be shown by adding of visible digits (in the figure the digits are shown in the thicken triangles).
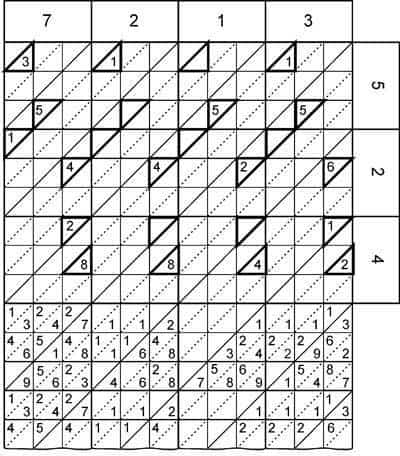
The next figure is an example of an inventor trying to improve upon Napier’s rods. The French physicist, cartographer, and engineer Pierre Petit (1594-1677), a King Counsellor and Intendant des Fortifications. Petit placed paper strips with Napier’s rods and made a mechanism, the so called Arithmetical Cylinder or Tambour de Petit, allowing the paper strips to be moved along the axes.
In late 1650s as a prominent mathematician, Kircher, was asked to prepare a set of mathematical tools for teaching the young Austrian crown prince (Archduke) Karl Joseph Erzherzog von Österreich (1649-1664). The order was fulfilled and a set of ten different tools of bone-like tablets for performing a variety of different tasks was manufactured and sent to the Archduke in 1661.
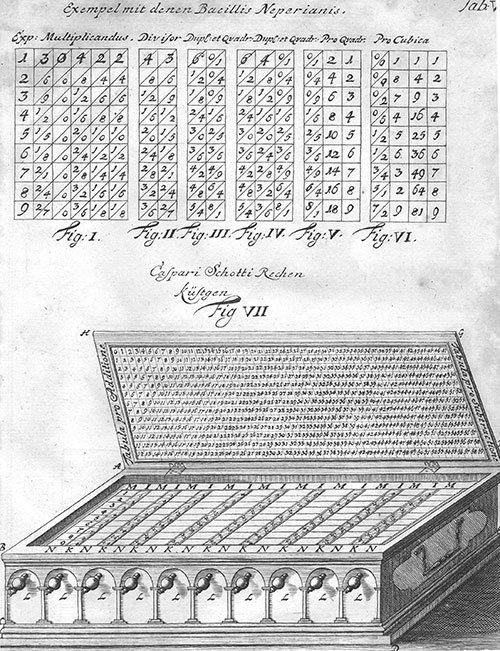
The toolset (called Organum Mathematicum, Mathematische Orgel or cista) was placed in a veneered wooden chest with a hinged lid and was described later in a book of his pupil and friend Gaspar Schott (1608-1666)—Organum Mathematicum libris IX explicatum, published in 1668 in Würzburg, Germany. The following is a photo of this device.

Later on the multiplication tool from organum mathematicum (with improvement of Schott) was described in Theatrum arithmetico-geometricum of Leupold. The arithmetic tool consists of ten cylinders, on which are placed strips with inscribed Napier’s rods. The cylinders are mounted in a box, which is closed to the upper side from a lined sheet of pasteboard with narrow vertical slits.
From the front side of the box are placed handles, attached to the cylinders, which can be used for rotating the cylinders and can be set with needed multiplicand on the rods. On the inside of the hinged lid of the box is inscribed an addition table to aid the operator.
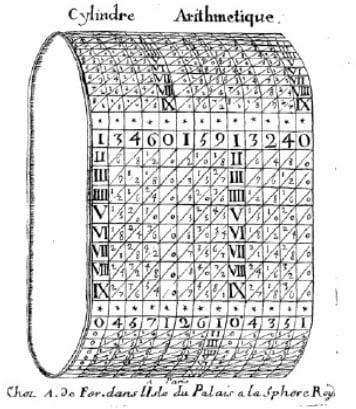
In 1673 cylindre artihmetique (Napier’s bones engraved on a cylinder) were used in the simple adding instrument nouvelle machine d’arithmétique of René Grillet de Roven
In the Theatrum arithmetico-geometricum of Leupold, already mentioned in this article, is described also a calculating tool, so called calculating drum, based on Napier’s rods (see the lower figure).
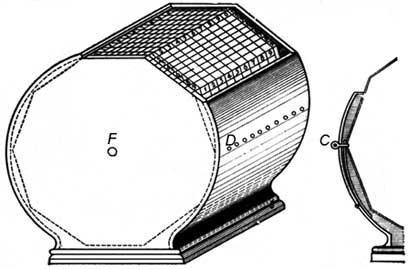
The following is a modern replica of the calculating drum of Leupold. This consists of 11 ten-sided disks, mounted on a common axes. The right side disk is fixed, while the remaining ten disks can be rotated with a hand. On the surface of the device are placed 10 round openings, which are used for fixing the angular position of the disks, by means of pins, pushed in the appropriate opening.
On the surface of each of the 10 sides of the rotating disk are inscribed the digits of the same Napier’s rod, while on the side of the fixed disk, with face to the operator, is inscribed a column of digits from 1 to 9. The multiplicand is entered by rotating the proper disks and fixing them by means of the pins against the fixed column of digits (multiplicands) of the fixed disk.

In 1728 the German scientist Johann Michael Poetius described in his book “Anleitung zu[r] arithmetischen Wissenschaft, vermittelst einer parallelen Algebra” (Instructions for arithmetic means of science, a parallel algebra) an instrument, composed of concentric moving circles (so called Mensula Pythagorica), which seems to be a variation of the Napier’s bones and can not render more services than the multiplication table (on the nearby image is shown a sector of Mensula Pythagorica).
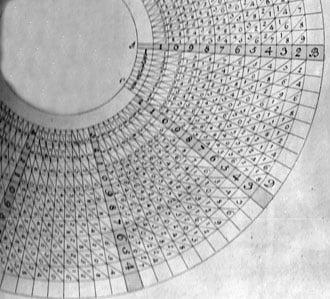
In 1789, the German mathematician M. Prahl devised an instrument, which he called Arithmetica Portatilis, and which was the same as the Mensula Pythagorica of Poetius, except that the movable circles were much larger and carried the numerals 1 to 100, so that with the aid of that instrument numbers could be added and subtracted up to 100.

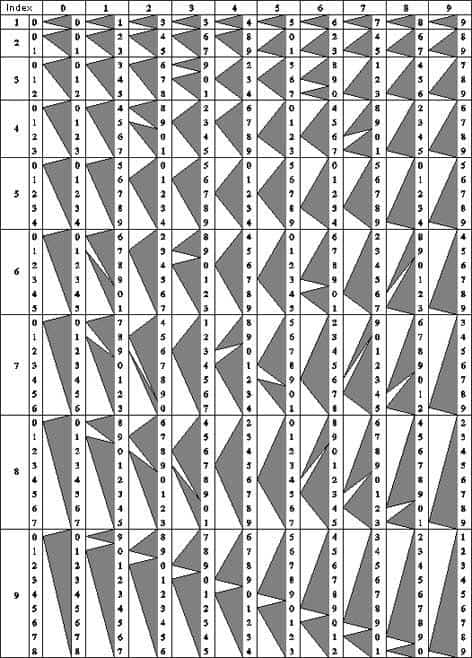
In 1885, the French mathematician—François Édouard Anatole Lucas (1842–1891) presented to the Académie Française a problem on arithmetic that caught the attention of Henri Genaille, a French civil engineer, employed by the railway system. Genaille, who was already quite well known for his invention of several different arithmetic aids, solved Lucas’s problem and, in the process, devised a different form of Napier’s rods.
This instrument eliminated the need to carry digits from one column to the next when reading off partial products. Genaille demonstrated his instrument (so called Réglettes multiplicatrices or in English Genaille-Lucas rulers) in 1891.
Napier’s Bones: Historical Significance
Napier’s Bones were influential because they influenced and inspired many other inventors to improve upon Napier’s Rods or come up with a similar device. Just one example is Charles Babbage. Babbage was a computer pioneer who worked on inventing the first programmable computer. This, in turn, led to more complicated mechanical designs.
By the 19th century, Napier’s bones were modified a bit to make them easier to use. The rods were then made with a 65 degree angle so that triangles that were used for adding were in alignment vertically. This meant that in each square of the rod, the ten or zero was on the left side while the unit is on the right. This made the components of each digit easier to read.
Napier’s rods and the lattice method of multiplication are still sometimes used in schools to help students learn to multiply and divide. Learning another method not only helps solve mathematical problems but helps expand critical thinking and give learners a new way to look at math.
Next Up…
The image featured at the top of this post is ©G-Stock Studio/Shutterstock.com.