Abacus Explained — Everything You Need To Know

Updated: July 25, 2023 by Rebecca Bales | Leave a comment

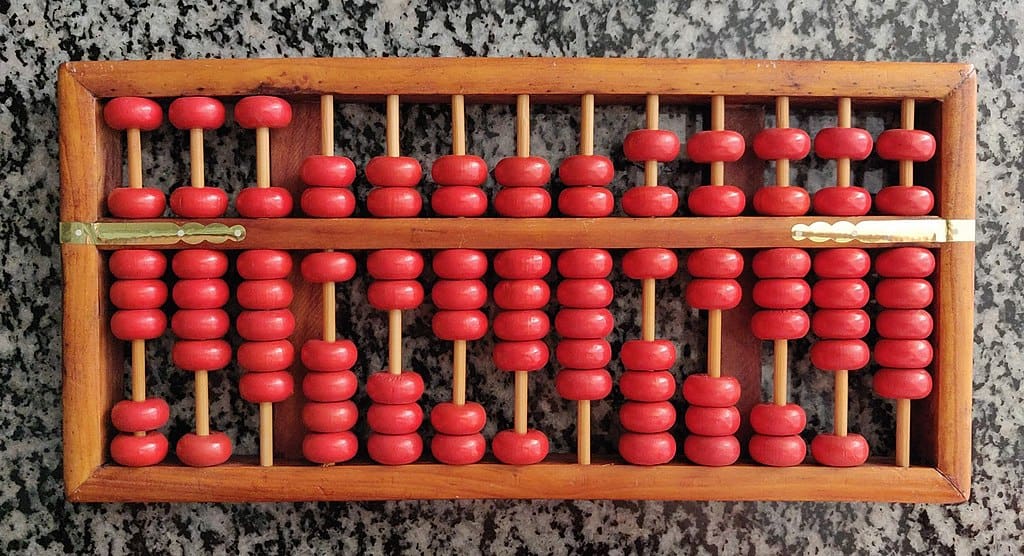
5 Facts About the Abacus

The origin of the abacus is still debated. Similar counting frame objects are believed to have been used in ancient China and Babylon.
An abacus is known as a Schoty in Russian and a Suanpan in Chinese.
Modern abacuses are used to assist students with visual impairments.
Using an abacus as a calculator has been shown to improve children’s mental math capabilities.
Modern computer operating systems use binary abacus to read numbers.

A roman abacus — This Roman abacus was made of metal and the beads move along slots.

The chinese Suanpan — The Chinese Suanpan is an abacus of Chinese origin.

The Soroban is an abacus of Japanese origin.

Abacus History

As the most ancient calculator known, the origin and inventor of the abacus is unknown. It’s been used for centuries in China and has a long history of use in Ancient Greece, Rome, Russia Japan, and Babylon.

The name is of Greek origin. Abax means “flat surface” in Ancient Greek. It was taken by the Romans and became abacus in Latin. Its pronunciation in English is “a-buh-kuhs.” This pronunciation stresses the first “a.”

Quick Facts

Created: Debated; Between 2,700 BC and 300 BC
Creator: Debated; Unknown creator in Babylonia or China
Original Use: Counting board
Cost: NA

Despite its ancient history, the abacus continues to be used in modern times. The modern abacus is attributed to Tim Cranmer, who invented the Cranmer abacus in 1962. It’s still used for teaching individuals who are blind or visually impaired.

The woman in this illustration is Typus Arithmeticae, considered the “muse of arithmeti.”

Abacus: How It Worked

Despite its long history and unknown inventor, the abacus has worked basically the same way throughout the centuries. It’s a fairly straightforward calculator that is still used in many countries in schools or markets for counting.

Most abacuses are made of a frame with wood rods and beads. Ancient devices used marble or stone parts. The idea of this counting frame is that each rod represents a sequential place value. Starting either with the tens place or a decimal place, increasing from right to left.

Many designs have four or five beads on a bottom row, with one to five beads on the top row. Pushing one bead from the top row to the center counts as five. You can then push additional beads from the bottom or, if available, from the top to count up to nine in that place value.

This counting frame allows individuals to track, add, subtract, multiply, and divide numbers easily. It helps ensure quick calculations when working with large numbers and makes the calculation process visible to both buyer and seller or teacher and student.

Abacus: Historical Significance

As commercial transactions became more complicated, a calculating tool was essential to make quick calculations and avoid errors. This origin, whether in Ancient China or Babylon, has been used throughout history and is continued to be used as a convenient calculator for commercial transactions.

The abacus is also an ancestor of the modern calculator and computer. Binary digit, the numbering scheme used to encode and decode digital messages, is based on an abacus design. The two possible binary digits are 0 and 1, but they are also described as low and high, which are the two possible positions for beads on an abacus.

The Cranmer Abacus

The Cranmer abacus is a modified version of the Japanese abacus or sorobon. This device was designed by Terence V. Cranmer in 1962. Shortly thereafter, the American Printing House for the Blind began selling the device, and they continue to sell it today.

This inexpensive, 13-rod abacus features a red felt backing which prevents beads from slipping during calculations. It measures 6 1/8” x 3 1/4” and the bead diameter is 3/8”. The device is considered to be a valuable teaching tool for visually impaired students. It can be used to perform addition, subtraction, multiplication, and division.

Cramer was blind from childhood. In his early years, he crafted and sold plastic jewelry. After being employed briefly at Kentucky Industries for the Blind, Cramer was a piano technician for 10 years. In 1952, he was employed by the Kentucky Division of Rehabilitation Services for the Blind. He was also a member of the National Federation of the Blind.

Cramer invented several devices, including a “Braille display switching matrix and circuit utilizing the same,” U.S. patent # 4,037,200.

The image featured at the top of this post is ©Jccsvq, CC0, via Wikimedia Commons.

Frequently Asked Questions

When was the abacus invented?

The exact date of the invention of the original counting frame is unknown. The abacus is believed to have been invented between 2,700 BC and 300 BC.

What is an abacus?

An abacus is a calculating tool that’s been used for centuries and is still popular in many countries. It’s pronunciation is “a-buh-kuhs.”

Who invented the abacus?

Because the abacus is one of the first calculators created, its origin may predate the historical record. The abacus inventor is unknown.

Where was the abacus invented?

The abacus was either invented in Babylon or in Ancient China. The exact history is unknown.

Who was the first to use the abacus?

The Babylonians, Ancient Chinese, Japanese and Russians all used a calculating tool similar to a modern-day abacus.

How do you use an abacus?

This calculating tool uses a counting frame and a series of beads on an upper and lower set of rods. Beads are pushed to the center to mark numbers in different place values, making it easy to make complex calculations.

Is the abacus the first computer?

While not technically a computer, the abacus is known as the first calculating tool. It’s also one of the first inventions that led to the first computer, credited to Charles Babbage in 1822.