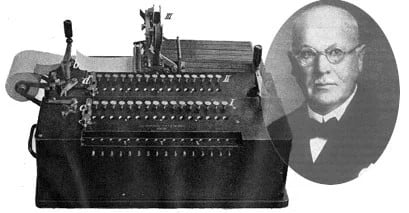
Christel Hamann’s 1909 Difference Engine: A Pioneering Mechanical Calculator

Christel Hamann’s difference engine was a type of mechanical calculator made in the 1900s. It was automatic and could help people calculate and arrange polynomial functions used in science, engineering, and navigation. Its name, “difference engine”, comes from the method it uses to do the math. The difference engine uses divided differences to arrange functions using a small set of polynomial coefficients. In other words, it could calculate many important tables of numbers, which was useful for the work of scientists and engineers.

3 Facts About Christel Hamann’s Difference Engine

• All its printed plates were given to Astronomisches Rechen-Institute in Berlin, and the first and second results were published in 1910 and 1911, respectively. 
• It was an invention built on the idea of two astronomers, Julius Bauschinger and Jean Peters, who wanted to do a further mathematical calculation using the tabulation method of known values with second differences. 
• Although it was a remarkable invention, Christel Hamann’s difference engine and its construction plan do not exist. What remains are pictures of the incredible mechanical calculator.

Christel Hamann’s Difference Engine History

The German engineer Christel Hamann (1870-1948) was a remarkable figure when it came to the invention of mechanical calculators. He is a holder of many patents in this area and the constructor of countless mechanisms and calculators such as Gauss, Berolina, Mercedes Euklid, Hamann Manus, etc.

It seems Christel Hamann owes his interest in the mechanics and construction of calculating machines to one of his father’s friends, namely the Würzburg Professor of Mathematics, Dr. Eduard Selling. In the 1880s Eduard devised a calculating machine of very original construction (see the machine of Selling), and the young Christel was allowed to take part in the building of this peculiar calculating machine. This collaboration with Professor Selling must be regarded as crucial for the later life of Hamann. Obviously from his youth, Hamann demonstrated an extreme talent and energy in techniques.

In 1905 The Royal Prussian Academy of Sciences in Berlin placed a considerable sum (15,000 Marks, later on, the Imperial Academy of Sciences in Vienna granted 8,000 Krones) at the disposal of German professors Julius Bauschinger (Director of the Imperial Observatory of Strassburg) and Jean Peters (professor, assistant of the Royal Astronomical Calculating Institute of Berlin), to prepare and publish tables of logarithms to eight digital places of all numbers from 1 to 200,000.

The work started using hand calculations in 1908, and after there was a need for an automatic mechanical calculator, the two astronomers contacted Christel Hamann. Bauschinger and Peters asked him to construct a new machine that would aid them in reckoning the values from the second difference by summation and finding correct results. 

Christel Hamann accepted their request, which marked the beginning of Christel Hamann’s difference Engine. He only needed a few months before the machine was ready early in 1909. 

The difference computing machine had great properties and weighed 40 kilograms (without the printing unit), was 145 centimeters wide, 20 centimeters high, and about 44 centimeters deep.

Also, the machine consisted of three parts: two similar independent calculating machines (adders) and a printing unit, which consisted in turn of a switch and a counter each. The first adder was placed next to the user, while the second difference was added to the first. The second added in the middle part created a sum added to the intermediate result. At the same time, the third section, which consisted of a printer, produced all the results done in the computing machine storage. All differences and the result can be set, operated, and printed up to sixteen places to increase accuracy. Additionally, each of the two adders is driven by its handle.

The first Tables of Bauschinger and Peters (Logarithmic-Trigonometrical Tables with eight decimal places from 1 to 20,000) were published in Leipzig in 1910, and the second publishing was done in 1911. Today, only one printed strip of the produced results and a beautiful picture of the amazing machine exists; unfortunately, all other assets can’t be traced.

Christel Hamann’s Difference Engine: How It Worked 

Hamann’s difference engine was used to simplify the polynomial calculations (mathematical functions involving different powers of the same variables), which normally would require all the arithmetic (addition, subtraction, multiplication, and division). 

The difference engine provides the first few polynomial values, and the other figures can be identified by adding a known value. For example, if the value of X is an incremental value of 1 in the table, it will show the values to be (1, 2, 3, 4.), which means the sequence will be adding on value as the calculation expands. Therefore, there is no need to keep checking on your calculator as it’s obvious the next polynomial figure will have 1 added value. 

However, the calculator could only perform one operation, limiting its production on cumbersome tasks. Nonetheless, it was a remarkable computing machine, as it could store data for future reference. It also had a printing system to produce printed strips of previous calculations.

Christel Hamann’s Difference Engine: Historical Significance

There existed other difference engines, created by prominent persons such as Charles Babbage (who created the first computing machine); however, Christel Hamann’s mechanical calculator is noteworthy as it was able to produce more accurate results for logarithms and trigonometric tables with 8-place figures. The figures could also be expanded to sixteen decimals to reduce errors in its solutions. 

Additionally, Hamann’s difference machine laid the foundation for building the interpolation method, which was used in the calculation. Christel Hamann made the difference machine a few months after being asked by Julius Bauschinger and Jean Peters.